This chapter gives you the Big Picture called cosmology. Cosmology is the study of the nature, origin, and evolution of the universe as a whole. The observational aspect of cosmology deals with finding distances to galaxies which is necessary for determining the geometry of the universe. This was covered in the last chapter. Vocabulary terms in the text are in boldface.
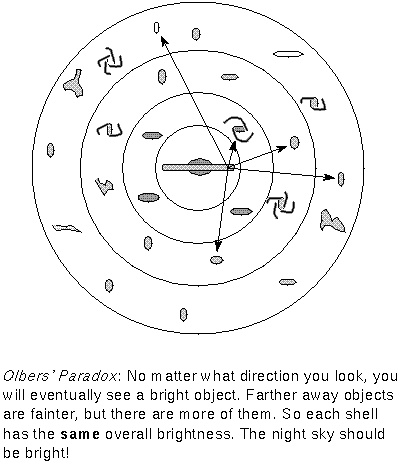
If the universe is uniformly filled with stars, then no matter which direction you look, your line of sight will eventually intersect a star (or other bright thing). Now it is known that stars are grouped into galaxies, but the paradox remains: your line of sight will eventually intersect a galaxy.

The brightnesses of stars does decrease with greater distance (remember the inverse square law) BUT there are more stars further out. The number of stars within a spherical shell around us will increase by the same amount as their brightness decreases. Therefore, each shell of stars will have the same overall luminosity and because there are a lot of ever bigger shells in an infinite universe, there is going to be a lot of light!
Any intervening material absorbing the starlight would eventually heat up and radiate as much energy as it absorbed, so the problem remains even if you try these ``shields''. Of course, stars are not points. They do have a definite size, so they can block light from other stars. The total brightness of the universe will not be infinite, but only as bright as the surface of a star (!). You can substitute ``galaxy'' for ``star'' in the preceding paragraphs if you want to update Olbers' Paradox for modern times. The way to resolve a paradox like this is to look at the assumptions that are used (the ``if'' statements) and determine whether or not they are valid.

The stretching of the light waves makes the light from galaxies appear redshifted, mimicking a redshift from the doppler effect as if the galaxies were moving through space away from us. However, the galaxies are simply being carried along with the expansion of the space between them---the whole coordinate system is expanding. The expansion of the universe means that galaxies were much closer together long ago. This implies that there is a finite age to the universe, it is not eternal. Even if the universe is infinite, the light from places very far away will not have had enough time to reach us. This will make the sky dark.
The Hubble law, speed = Ho × distance, says the expansion is uniform. The Hubble constant, Ho, is the slope of the line relating the speed of the galaxies away from each other and their distance apart from each other. It indicates the rate of the expansion. If the slope is steep (large Ho), then the expansion rate is large and the galaxies did not need much time to get to where they are now. If the slope is shallow (small Ho), then the galaxies need a lot of time to get to where they are now.
The age of the universe can be easily estimated from the simple relation of time = distance/speed. The Hubble Law can be rewritten 1/Ho = distance/speed. Notice that the expansion time interval = 1/Ho. The Hubble constant tells you the age of the universe, i.e., how long the galaxies have been expanding away from each other: Age = 1/Ho. This value for the age is an upper limit since the expansion has been slowing down due to gravity. That means that the Hubble ``constant'' actually was larger in the past. Taking the expansion slowdown into account, you get an age closer to 2/(3 Ho). Still, the age looks like a number × (1/Ho), so if the Hubble constant is large, the derived age of the universe will be small.
The idea of a uniform universe is called the cosmological principle. There are two aspects of the cosmological principle:
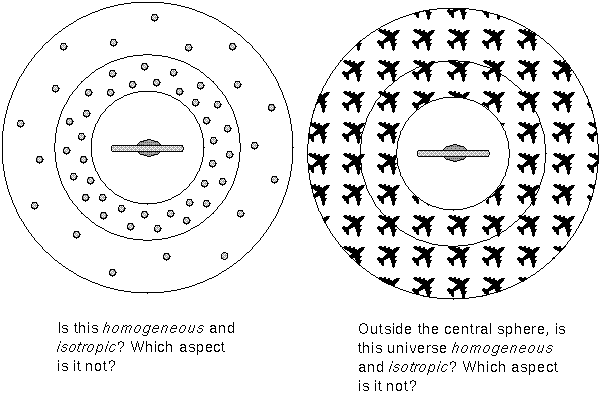
The cosmological principle is a Copernican idea. It means we are not in a special place. Every observer at a given cosmological time will see the same thing, such as the same Hubble law. ``Cosmological time'' in this context means the time measured from some common event like the creation of the universe. Everyone at the same cosmological time will measure the same age of the universe. The cosmological principle allows the universe to change, or evolve, throughout time.
An extension of the cosmological principle called the perfect cosmological principle says that the universe also does not change with time; there is no evolution. Therefore, in an expanding universe, new matter must be continually created. This violates a central rule of nature known as the law of the conservation of mass. This law says that the total amount of mass does not change---mass is not created from nothing or destroyed. However, the amount of new matter that would need to be created for the perfect cosmological principle to be true is quite small---only one hydrogen atom per cubic centimeter every 1015 years. This is approximately one hydrogen atom/Houston Astrodome every year---a very small amount! As described in the last chapter, the increase in the number of quasars at large distances from us, is strong evidence of a universe that DOES change, or evolve. Other evidence for a changing universe is given later in this chapter.
To help understand what curved space means, let's use the analogy of a two-dimensional world curving into the third dimension. Pretend you are confined to the surface of a balloon and you only know about ``front'', ``back'', ``left'', and ``right'', but not ``up'' and ``down''. In your 2D universe you cannot see the third dimension. Your universe appears flat. Yet you know that your 2D universe must be curved because if you walk in a straight line, you eventually arrive back at where you started! The balloon universe has a finite size but no edge. You also know that the angles of large triangles add up to a number larger than 180ƒ! For example, on the balloon the lines of longitude running north-south intercept the equator at a 90ƒ angle and converge at the poles. So a triangle made of one point on the equator + the north pole + another point on the equator will have the angles add up to more than 180ƒ. In a truly flat universe, the angles would add up to exactly 180ƒ. You would be able to deduce that your universe is positively curved.
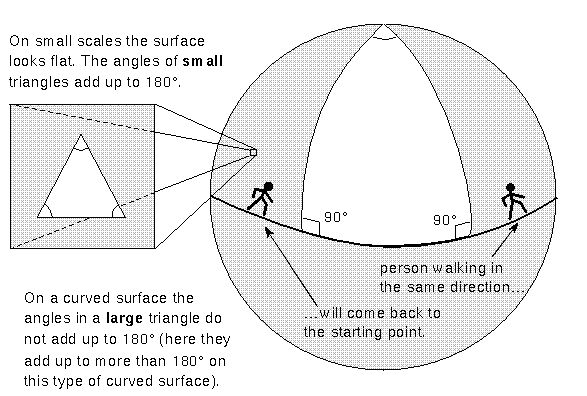
On sufficiently small scales the surface looks flat so the regular geometry rules apply. The angles in a small triangle add up to 180ƒ. Here on the surface of the Earth, the Earth looks flat to us because the curvature of the Earth is so much larger than we are. The universe does not have to curve back on itself as shown in the illustrations above. This type of positively-curved universe is usually easier to picture, but the curvature could be the opposite. In a negatively-curved universe, the universe curves away from itself. A two-dimensional analogy would look like a saddle. The angles in large triangles would add up to less than 180ƒ. Like the positively-curved universe, there would be no center on the surface and no edge.
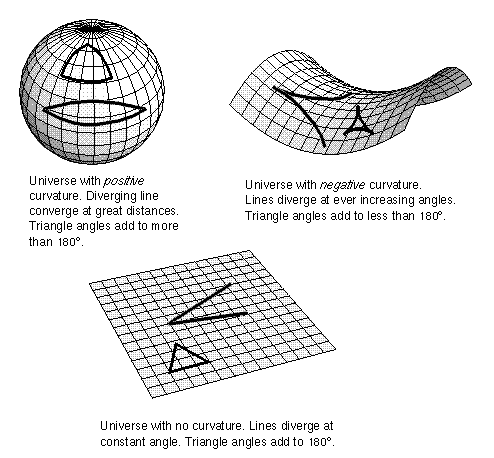
Rather than setting up BIG triangles in the universe, astronomers can use how the number of galaxies increases with increasing distance. If the universe has zero curvature and the galaxies are spread roughly uniformily in the universe, then the number of galaxies should increase linearly with ever greater volume. Lines defining an angle spread out in straight lines. If the universe has positive curvature, then the number of galaxies increases with greater volume then decreases with very large volumes. Lines defining an angle spread out at first and then converge at great distances. If the universe has negative curvature, then the number of galaxies increases more rapidly with with ever greater volume than a flat universe. Lines defining an angle diverge at increasing angles as the lines curve away from each other.
The idea of a curved surface also explains why astronomers in every galaxy will see the other galaxies moving away from it and, therefore, derive the same Hubble Law. Go back to the balloon analogy, imagine that there are flat houses on it. As the balloon expands, the elastic material moves the houses apart from each other. A person sitting on their front porch see everybody else moving away from her and she appears to be the center of the expansion.
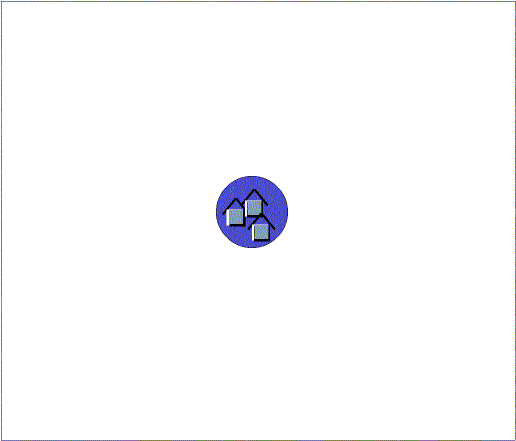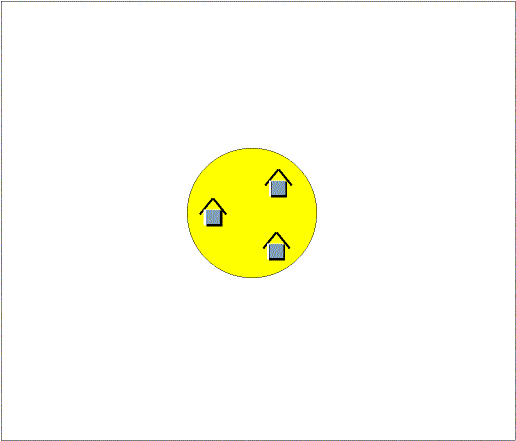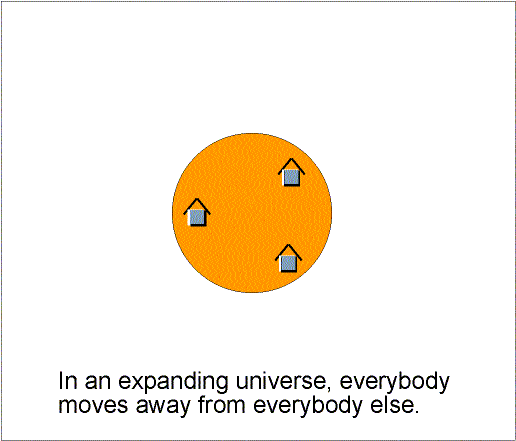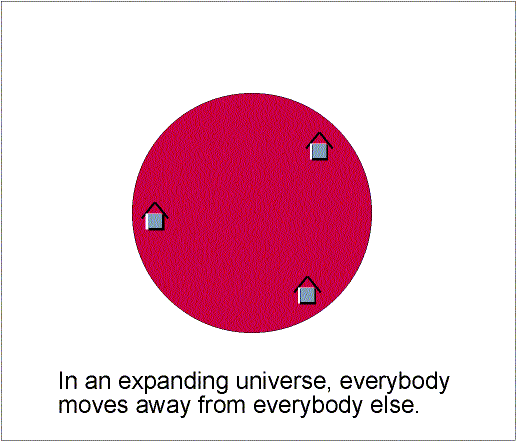
Now add another dimension and you have our situation. Just like there is not new balloon material being created in the 2D analogy, new three-dimensional space is not being created in the expansion. Like any analogy, though, the balloon analogy has its limits. In the analogy, the balloon expands into the region around it---there is space beyond the balloon. However, with the expanding universe, space itself is expanding in three dimensions---the whole coordinate system is expanding. Our universe is NOT expanding ``into'' anything ``beyond''.
| cosmological principle | cosmology | homogeneous |
|---|---|---|
| Hubble constant | Hubble law | isotropic |
| Olbers' Paradox | perfect cosmological principle |
![]() Go to
cosmic microwave background section
Go to
cosmic microwave background section
last update: 05 April 1999
(661) 395-4526
Bakersfield College
Physical Science Dept.
1801 Panorama Drive
Bakersfield, CA 93305-1219