
Just like you found for the determination of stellar properties, finding the distance to the galaxies is essential for comparing the galaxies against each other. In order to determine the luminosities and masses of the galaxies and the distribution of the mass inside the galaxies, you must first know the distances to them. Is that galaxy in our telescope bright because it is producing a lot of energy or because it is close to us? Are the stars moving quickly in a galaxy because the combined mass is large or because the distances inside the galaxy are small? You need to find the distance to the galaxy to be sure.
One method of determining the distance discussed above uses the period-luminosity relation of Cepheid variable stars to derive the distance from the apparent brightness of the Cepheids. This works for the nearby galaxies. To find the distance to galaxies farther away, other standard candle techniques involving objects more luminous than Cepheids like supernova explosions or supergiant stars are used. Unfortunately, their luminosities are not as well known or uniform, so there is greater uncertainty in the derived distances to the very distant galaxies.
Another method uses the width of a galaxy's spectral lines. The width of the absorption lines from the stars or the radio emission lines from the gas in a galaxy depend on the amount of stellar motion in the galaxy. Most galaxies are too far away for us to resolve the fine-scale details of the internal motions in the galaxies. The spectrum of far away galaxies is from the combined motions of all of the material in them.

Some stars in a far away galaxy are moving toward us, so their absorption lines will be blueshifted. Other stars will have redshifted lines because they are moving away from us. The resulting spectral lines from the entire galaxy blend together to form a FAT line. The same effect is seen with the gas motions (caused by heat and rotation) inside a single star or gas cloud in our galaxy. The more random motion there is in an elliptical galaxy, the greater the spread in the distribution of velocities and the fatter the resulting galaxy spectral lines will be. More massive galaxies have more gravity so their stars are accelerated to faster orbital speeds. More massive galaxies should have broader lines and if the luminosity correlates with the mass, the more massive galaxies should have greater luminosity.
Sandra Faber and Robert Jackson discovered in 1976 a simple relation between the spread of velocities (called the velocity dispersion) and the luminosity of elliptical galaxies. For the inner few kiloparsecs of the elliptical galaxies, the velocity dispersion = 220 × (L/L*)0.25, where L* is a characteristic galaxy luminosity (around 10 billion solar luminosities). This is known as the Faber-Jackson relation. An elliptical galaxy's luminosity can be found from the velocity dispersion of the stars in the central few kiloparsecs of the galaxy.

In 1977 Brent Tully and Richard Fisher discovered a similar relation between the rotational velocity of the disk and the luminosity of a spiral galaxy. The rotational velocity is found from the 21-cm emission of the neutral atomic hydrogen gas in the outer parts of the disk. The rotation curve is flat in the outer parts of most galactic disks (dark matter!). The part of a spiral galaxy's disk that is rotating toward us will have its lines blueshifted. The part of the disk rotating away from us will have its lines redshifted. The 21-cm emission from a galaxy of small angular size will be the blended result of the emission from all parts of the disk. The faster the disk rotates, the broader the 21-cm emission line will be. The Tully-Fisher relation for the infrared luminosity is: circular velocity = 220 × (L/L*)0.22. Infrared is used to lessen the effect of the dust in our galaxy and in the other spiral galaxy. The luminosity of the galaxy is found from the width of the 21-cm emission line and the distance is then derived using the apparent brightness and the inverse square law. The methods of determining the distances to the galaxies are summarized in the ``Steps to the Hubble Constant'' section.

If the distance is measured in units of megaparsecs (Mpc) and the recession speed is in kilometers/second (km/sec), the Hubble constant (slope of the line) is between 75 and 85 km/sec/Mpc. This value is found by using the galaxies that have accurate distances measured (Cepheids, etc.) and dividing their recession speeds by their distances. It is easy to find the recession speeds of galaxies from their redshifts. The Hubble law provides an easy way to measure the distances to even the farthest galaxies from the (recession speed/H). For example, if a galaxy has a redshift of 20,000 km/sec and H is set to 75 km/sec/Mpc, then the galaxy's distance = (20,000 km/sec)/(75 km/sec/Mpc) = 20,000/75 × [(km/sec)/(km/sec)] Mpc = 267 megaparsecs.
At first glance it looks like the Milky Way is at the center of the universe and it committed some galactic social blunder because all of the other galaxies are rushing away from it (there are a few true galactic friends like the Andromeda Galaxy that are approaching it). The Hubble Law shows that there is actually not a violation of the Copernican principle. Because the more distant galaxies move faster, this means that the galaxies (or galaxy clusters) are all moving away from each other---the universe is expanding uniformily. Every other galaxy or galaxy cluster is moving away from everyone else. Every galaxy would see the same Hubble Law.

In the next chapter you will see that the expansion of the universe is the expansion of space itself---the galaxies are not moving through space, but, rather, they are being carried along with the expansion of space. For now, use the figure above to see how a uniform expansion gives us the Hubble Law. Since space itself expands, all distances will increase by the same factor in the same amount of time. Galaxy (a) is 10 Mpc from galaxy (b) and galaxy (c) is 10 Mpc from galaxy (b). If the Hubble constant this time is 77 km/sec/Mpc, then galaxy (a) sees galaxy (b) moving at 770 km/sec away from it and galaxy (c) is moving away from galaxy (b) at 770 km/sec. Astronomers on galaxy (a) will see the galaxy (c) moving away at (770 + 770) km/sec = 1,540 km/sec, twice as fast as galaxy (b), because galaxy (c) is at a distance of (10 + 10) Mpc from galaxy (a), twice as far as galaxy (b).
The galaxy (a) astronomers see the other galaxies moving away from them and their home galaxy appears to be at rest. Now jump to galaxy (c). To the astronomers on galaxy (c), galaxy (a) is moving away at 1,540 km/sec and galaxy (b) is moving away at 770 km/sec. Their home galaxy (c) appears to be at rest. You can use similar reasoning to show that everybody will also get the same numerical value for the Hubble constant. The figure above also illustrates another point about the expansion. Notice that the sizes of the dots and the size of the threesome group at center-top do not change. The galaxies themselves do not expand and the galaxies inside a cluster do not expand away from each other. On small size scales, the internal gravity of a galaxy or galaxy cluster prevents the expansion inside the galaxy or galaxy cluster. The Hubble Law applies for only the very large distances.
Another example of why everyone will see the same Hubble Law is given in the figure below. Each of the galaxies in the figure is 100 million parsecs (about 300 million light years) apart from each other and the Hubble constant is set to 75 km/sec/Mpc here. If you choose galaxy C to be your home galaxy, then you will see galaxies B and D moving at 7,500 kilometers/second away from you because they are at a distance of 100 megaparsecs from you. Galaxies A and E are 100 megaparsecs from their closest neighbors, so they are moving away from them at 7,500 kilometers/second and 15,000 kilometers/second away from galaxy C since they are each 200 megaparsecs from galaxy C. What would the galaxy speeds be if you chose galaxy D or galaxy E as your home galaxy?

For spiral galaxies the rotation curve is used to measure their masses like is done to find the mass of the Milky Way. The rotation curve shows how orbital speeds in a galaxy depend on their distance from the galaxy's center. The mass inside a given distance from the center = (orbital speed)2 × (distance from the center)/G. The orbital speed is found from the doppler shifts of the 21-cm line radiation from the atomic hydrogen gas. The angular distance of the piece of the disk from the center, but to use the enclosed mass formula, the the piece of the disk's actual linear distance from the center of must be found.
Remember way back in the planetary science chapter that the linear distance can be found from the angular distance if you know the distance to the object? The linear distance from the galaxy center = [(2p × (distance to the galaxy) × (angular distance in degrees)] / 360ƒ. This is why you must first know the distance to a galaxy if you want to measure its mass.
For elliptical galaxies the width of the absorption lines from all of the stars blended together is used to measure the mass of elliptical galaxies. The width of the absorption lines depends on the spread of the distribution of the velocities---the velocity disperion. The elliptical galaxy's mass = k × (velocity dispersion)2 × (the distance the stars are from the galaxy center)/G, where k is a factor that depends on the shape of the galaxy and the angle the galaxy is from Earth.
The stars and gas in most galaxies move much quicker than expected from the luminosity of the galaxies. In spiral galaxies, the rotation curve remains at about the same value at great distances from the center (it is said to be ``flat''). This means that the enclosed mass continues to increase even though the amount of visible, luminous matter falls off at large distances from the center. In elliptical galaxies, the gravity of the visible matter is not strong enough to accelerate the stars as much as they are. Something else must be adding to the gravity of the galaxies without shining.
That something else is called dark matter. It is material that does not produce detectable amounts of light but it does have a noticeable gravitational effect. Astronomers are not sure what the dark matter is made of. Possibilities range from large things like planets, brown dwarfs, white dwarfs, black holes to huge numbers of small things like neutrinos or other particles that have not been seen in our laboratories yet. The nature of dark matter is one of the central problems in astronomy today.
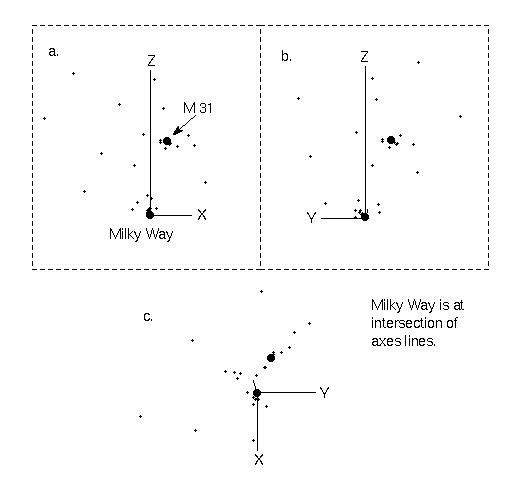
Our Milky Way is part of a poor cluster called the Local Group (an imaginative name, yes?). The Local Group has two large spirals, one small spiral, two ellipticals, 13 irregulars, and 14 dwarf ellipticals. There may be more irregular and dwarf ellipticals. The distribution of the galaxies is shown in the figure below. The Local Group is about 3 million light years across with the two large spirals, the Milky Way and Andromeda Galaxy, dominating the two ends. Each large spiral has several smaller galaxies orbiting them. The proportions of the different types of galaxies in the Local Group probably represents the number of the different types of galaxies in the rest of the universe. The small galaxies can be seen in the Local Group because they are close enough to us. But the dwarf galaxies are hard to see in far away clusters.
The closest large cluster to us is the moderately-rich cluster called the Virgo Cluster because it is in the direction of the Virgo constellation. It has many hundreds of galaxies (mostly spirals and irregulars) distributed into an irregular shape about 10 million light years across. It is about 49 to 59 million light years from us. Some ellipticals are present in the central part of the cluster including a giant elliptical at the center (M87) that has become so large by gobbling up nearby galaxies that were attracted by its enormous gravity. The total mass of the Virgo cluster is large enough that its gravity pulls nearby groups of galaxies (including the Local Group) toward it.
A rich cluster is seen in the Coma constellation---the Coma Cluster. It has thousands of galaxies (mostly ellipticals and S0 spirals) in a large, spherically-shaped cluster about 300 million light years from us. It is at least ten million light years across. The elliptical galaxies congregate toward the central regions while the few spirals are found on the outskirts. Two giant ellipticals (NGC 4874 & NGC 4889) occupy the central part of the cluster. Like M87 in the Virgo Cluster, they have grown very large from pulling in galaxies that were unfortunate to have strayed too close to escape the giant ellipticals' gravity. Other rich clusters show the same segregation of the spirals from the ellipticals as the Coma Cluster. Ellipticals gather together in the center of clusters while spirals prefer to stay close to the periphery.
Two pioneers in the mapping of the structure of the universe are Margaret Geller and John Huchra. They and their students have taken thousands of spectra of galaxies along thin pie-shaped slices of the sky. A recent map of theirs is shown on below. It would take much too long to take spectra of galaxies in every direction in space, so they have chosen to map the universe in slices. The slice map below took about 15 years of data-collection to generate! Geller and Huchra's have shown us surprising results about the large-scale structure of the universe. Other groups of astronomers have joined their efforts to map the universe.

The arrangement of the superclusters and voids looks like a bunch of soap bubbles or swiss cheese with the galaxies on the borders of the huge holes. What produces the long thin strands of clusters around the huge bubbles of empty space? Obviously, gravity is the force at work, but how has it worked to produce these structures? Dark matter must play a significant role but how it does that is not known. Astronomers are using powerful supercomputers to simulate the gravitational interactions of millions of particles and they program guesses of the behavior of dark matter into the simulation code. Early results from the simulations are able to produce the filamentary structure and voids.
The picture below shows a supercomputer simulation of a 300 million light year cube of the universe using 47 million particles interacting with each other through the force of gravity. The galaxies clump together into clusters and the galaxy clusters gather together into huge string-like superclusters with big gaps (voids) in between. The colors represent differences in density: from lowest to highest density the colors are black, blue, pink, red, orange, yellow, and white (highest density peaks). The image is a much compressed jpeg version of a gif image from the HPCC group at the University of Washington. Some details have been lost in the compression, but you can select the image to bring up the original uncompressed gif file.
![]() Go to
Introduction and galaxy types sections
Go to
Introduction and galaxy types sections
![]() Go to
Galaxy Origins and Active Galaxies sections
Go to
Galaxy Origins and Active Galaxies sections
last update: 06 May 1999
(661) 395-4526
Bakersfield College
Physical Science Dept.
1801 Panorama Drive
Bakersfield, CA 93305-1219